Progressive power lenses - part 1

Progressive power lenses are designed to provide continuous vision at all distances instead of the predetermined working distances of a bifocal or trifocal design. A progressive lens can be considered to have three zones, just like a trifocal lens; a distance zone, a progression zone, and a near zone (figure 1). Unlike a trifocal lens, however, the power of the lens increases over the progression zone from the distance power at the top to the near power at the bottom (figure 2).

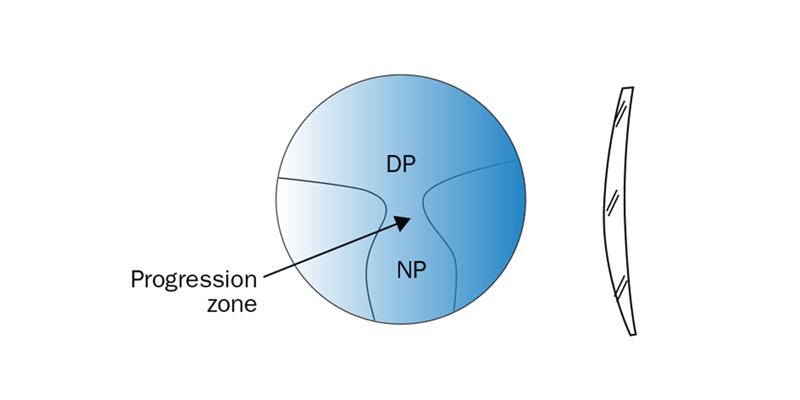
Figure 1: Progressive power lens

The difference in power distribution between an E-style trifocal lens and a progressive power lens is shown by the graphical plots in figure 2, which indicate how the power varies from the top to the bottom of each type of lens. The variation in power along the vertical meridian of the lens is usually illustrated graphically, as shown in figure 2. The two graphs compare the discrete power areas of a trifocal lens (2(a)) with the gradual increase in power of a progressive lens (2(b)) from distance to near. The term power variation lens is sometimes used to describe these lenses.